A discussion about Tungsten Cored Ammunition (APCR/HVAP, and APDS)

[KillaKiwi]

Man, imagine special ammunition being actual effective, worth using and limited instead of spamming the best round like they built nothing else.

It also reminds me of air battles. The games been out for 11 years and they never bothered to add missing ammo types, like the HEI-T for Italian 12.7mm or APHE for MK 101/103s.

But now we can drop napalm on bases and use AP bombs that probably no one botheres to use.

I think I can see a pattern 🤔
If it’s a module to unlock that pays with money or playing time, regardless how useless it is, its worth implementing.
If that’s not the case, Devs don’t bother.

For Gaijin to feel compelled to make any changes, they probably need to make each ammo belt unlockable instead of all at once.
Then they will be like: Here are 5 new ammo types you can unlock.

[Conraire]

One of the 3-4 Major version revisions of 90/40mm T320 ;). The version in game now, I believe is modeled after T320E10, which used an all steel penetrator.

A Diagram ;)

[SpardaSon21]

@Peasant_wb

Also, making these shells limited in quantity is in line with historic reality.

Depends on the time frame, really. APCR was standard for the USA by Korea. Turns out that the richest country in the world has no problems firing wads of cash out of their tank guns instead of more conventional rounds.

[KillaKiwi]

Well, as tank armor got more effective it also become more expensive to deal with them :)

[SpardaSon21]

True, but even in Korea when the T-34/85 was the primary medium tank that the 90mm would have had no problems with we still shot APCR at them.

Of course if you were a Sherman crewman APCR was a godsend. Over 180mm of pen in the close fighting typical to the Korean mountains would let you blow a hole clean through any part of it.

[Peasant_wb]

People often assume that tungsten carbide projectiles are quite simply not susceptible to shatter, unlike the normal steel AP. It’s a widely held… misconception.

The truth is quite different:

The British report, EFFECT OF IMPACT AND VELOCITY, listed the results of APDS testing for shatter velocity, which was found to vary with angle.

Tungsten projectile shatter failure is based entirely on angle and velocity, as follows:

Impact Angle…critical velocity
0…4132 fps
5…3849
10…3792
15…3528
20…3472
25…3377
30…3321
35…3283
40…3264
45…3283
50…3340
55…3472
60…3623

You can observe this happening here in the velocity range predicted by british data. For lower hardness plate hits at around 3000fps don’t shatter but once they do the ballistic limits jumps upwards suddenly.

source: Shatter gap in uncapped AP shot. - Historical Discussion - War Thunder - Official Forum

[Peasant_wb]

Hey look what I found: the penetration for the 85mm BR-365P projectile differs in this table when compared to this other (later one).

Spoiler

Spoiler

Source: Firing Tables for the 85mm guns, 3-rd edition 22 February 1945.

Edit: interesting note:

“In this edition … is ascertained the armor penetration of the BR-365 and BR-365P projectiles and was added back the info on armor penetration of the BR-365K projectile”

Interesting. Why have they removed the data on pen of this shell from previous edition? Because from the context it sounds like it was present in the first edition of these tables.

CIA-RDP83-00418R001800060007-8.pdf

[Peasant_wb]

@Peasant_wb

That’s… actually a good point. I wasn’t considering the possibility that a different relative mass of the core carrier might change the ballistic limit in a significant way.
If your theory is correct, the APCR shells with higher fraction of their mass in the carrier would perform better, relative to the absolute mass of their tungsten cores.

I will look into this.

I did look into it. :)

Now I’m back to share my findings: I have confirmed that the mass of the steel sheath(APDS)/carrier(HVAP) does indeed increase the armor penetration when compared to the core alone.

I have also developed a mathematical model to account for this effect.

Edit: Interestingly, if we try to apply this model to full caliber shells, lets say the 88mm Pzgr.39/43, and use the weight of the shell w/o cap and windshield (8.8kg) and set the contribution from the carrier to zero, we get pen of around 230mm/0° at 100m.

Edit:

Formula

Details

Graph

Edit: Change K_0 to 1880 and U to 210 and n to 1.26

Edit: pls someone comment in this thread, the forum doesnt let me post more than 3 comments in a row.

Anyway, if this game will ever implement face-hardened armour, it will be only fair to properly model the soviet high-hardness armour as well.
And it’s much stronger against APCR than normal hardness RHA.

[Kmdo_Peach]

Very interesting formula, here’s your reply.

[Peasant_wb]

Abstract

Schematic

Graph

Obliquity Shatter gap

Link: https://apps.dtic.mil/sti/tr/pdf/ADA800130.pdf

These findings broadly match the information I’ve posted earlier.

Spoiler

A discussion about Tungsten Cored Ammunition (APCR/HVAP, and APDS) - #217 by Peasant_wb

[Peasant_wb]

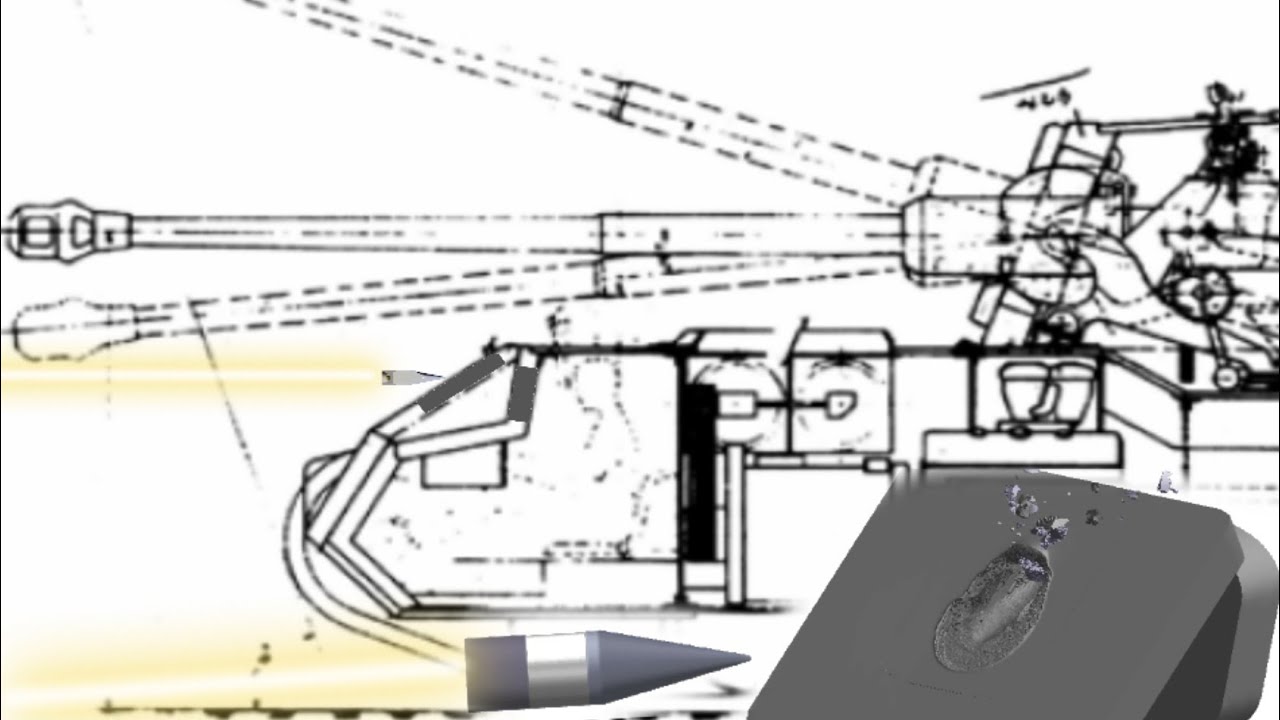
I’ve made a 3D model of the HVAP carrier for the 76mm M93.

Spoiler

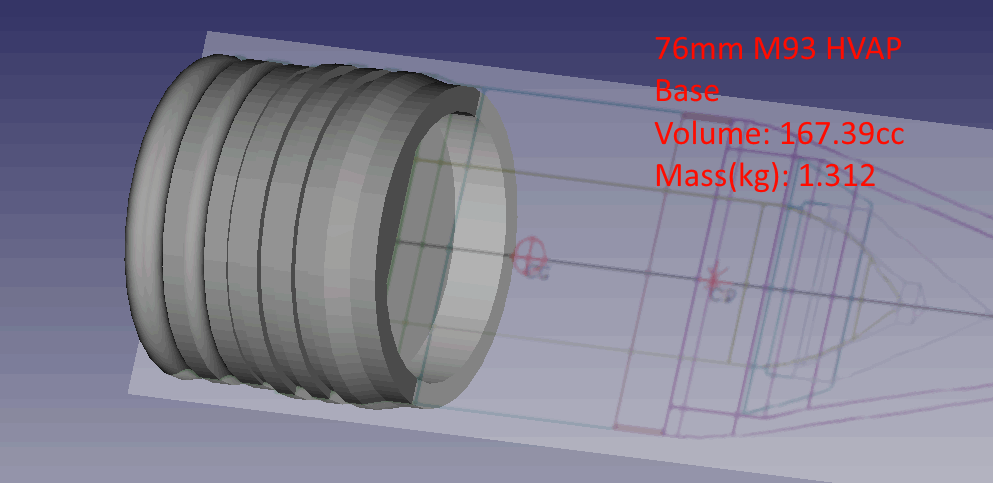
As I was looking at it, a thought came to my mind. Let me show you something first:

Here is the tungsten core for the 76mm HVAP:

Spoiler

It’s exactly 5in. long. The body from the base to the bourellet is about 4in long. Which means for vertical armour plates thinner than 4in. the core will perforate them before the base impacts the armour, assuming they stay together during penetration process, like this:

Spoiler

if its true, then for plates around 4in. and thinner, the ballistic limit for this shell would be as for an equivalent projectile with the total mass equal to that of the core AND the steel base, but diameter of only the core.

Now let’s calculate the two and compare them to historical data:

Spoiler

What do we see? The historical ballistic limits for 4 and 5in. plates at 0° are much closer to those that we get by assuming that the core penetrates without any additional help from the steel base.

Which implies that this is the case IRL. We don’t know for sure, but its a safe bet to assume that on impact with the armour the cylindrical base of the tungsten core applies so much force to the steel base of the HVAP projectile, that it shears a steel disk out of the base and it no longer contributes any kinetic energy towards the penetration of the armor.

Now, the historical ballistic limits are still a bit lower than my estimates for a naked core, which suggest that the carrier still contributes a little bit of kinetic energy to penetration, but a much smaller amount than previously thought.

I apologise to all people who came to this forum to talk about a videogame and not to read a lecture on physics. :)

Edit:

@KillaKiwi

But you’re comparing arrowhead APCR rounds with a large steel sheath against the performance of just a tungsten core.
For the 45mm APCR the penetrator makes less than a 1/3 of the shell weight.
Obviously the steel sheath can not be entirely ignored.

@KillaKiwi I believe that the higher than expected ballistic limit in that trial with .40in tungsten core is due to excessive projectile yaw. It is much more detrimental to penetration of subcaliber projectiles than full caliber ones. My estimates still match very closely to every other 0° APCR testing data I have. It gives the expected BL of 2762fps against 1.5in./0° RHA of 300 Brinell.

[Conraire]

So, I’ve been tinkering with the 2C6 Nomograph calculator recently. I figured out how much of the carrier mass from M304 is applied to the core during low obliquity angle penetration, and have it calibrated to match historic penetration results at firing table velocities for the M304/T30E16 round at 220bhn. If it’s not exactly on for the round, it’s within 1-2mm for the range table velocity.

It’s also quite useful, in the fact that once its calibrated to a specific round design. It can be used to determine a velocity table/curve for a round or rounds where little or no information is available, as long as there is some form of historic penetration data to go by.

For example, the T30E16 based 90mm HVAP rounds. Which would be M304(90mm M3 Gun), T44(90mm T15 Guns), and T44E2(90mm T54 Gun), all basically use the same 8lb core and base carrier design. The only differences may be in driving band placement. However, they all fire the rounds at different muzzle velocities.

90mm M3 Guns

• M304 = 3350ft/s or 1021m/s

90mm T15 Guns

• T44 = 3750ft/s or 1143m/s

90mm T54 Gun

• T44E2 = 3875ft/s or 1181m/s

The constant with the T30E16 design is of course the as fired mass of 16.8lb or 7.62kg, 8lbs/3.628kg of which is the core, an unknown amount is the Steel base cap and tracer assembly, and the rest is the aluminum carrier and windshield. After subtracting the 8lbs from the core, that leaves 8.8lbs/3.992kg of carrier mass.

During calibration to M304, I found that, in order for the round to meet its historic performance levels at firing range table velocities, a penetration mass of 8.686lb/3.94kg was required. Which would mean, about 0.688lb/312g (7.81%) of the carrier mass are acting on the core, during initial penetration. Most of that is likely from the steel base section acting as a piston pushing on the core.

I’m not at home near my PC at the moment, so I’ll have to elaborate more later.

[KillaKiwi]

@Peasant_wb

Which implies that this is the case IRL. We don’t know for sure, but its a safe bet to assume that on impact with the armour the cylindrical base of the tungsten core applies so much force to the steel base of the HVAP projectile, that it shears a steel disk out of the base and it no longer contributes any kinetic energy towards the penetration of the armor.

Now, the historical ballistic limits are still a bit lower than my estimates for a naked core, which suggest that the carrier still contributes a little bit of kinetic energy to penetration, but a much smaller amount than previously thought.

When estimating the performance of the APCR carrier, I used to just consider the steel weight behind the core. Both for simplicity sake but also since I knew that the total weight couldn’t be used, since not the full KE of the carrier would be transfered the core.

Seems like the approach works decently well.

[Conraire]

I think this simulation video by Dejmian is probably the best way to visualize it.

Dejmian M93 HVAP Sim

(https://youtu.be/HJ1d-W_M9wE?si=AeNPC3XiEdi6Hb3f)

As you can see, during the initial penetration impact phase, the steel base section stays intact, pushing on the core until it hits the plate surface. And then a small portion of the carrier base breaks off and follows the core through the hole.

On the other hand, that also somewhat explains why those earlier HVAP/APCR rounds would be worse against sloped armor than an APDS round. As at angles above ~50-55° that base section would get deflected off the base off the core much sooner.

I believe the later rounds like M319, and M332 also benefit somewhat from the base piston effect as well, due to how the carrier is designed, even though the base is aluminum alloy.

[FlipAllTheTables]

Dejmian also made this simulation, using a supercharged M93 round versus a hypothetical armor set up of the Ferdinand using slopped spaced armor rather than 2 plates of armor stuck together.

Supercharged 76mm M1A2 vs Ferdinand armor version | Armor Penetration Simulation

One thing I want to point out is that according to him these armor plates are 80 mm at 55 degrees followed by 100 mm at 9 degrees. M93 just about managed to penetrate the first plate.

Obviously, this is just a simulation, so it’s not proof of anything, and this is being fired at a supercharged velocity. But I do remember something about the British figuring out that 17 pounder APDS needed over 3410 ft/s (1040 m/s) of velocity to defeat a Panther upper glacis. It seems that M93 follows that closely (and M93 has a very similar core to 17 pounder APDS, only slightly larger by 0.5 mm and heavier by around 0.02 kg) .

It could be that late war US APCR rounds follow a slope armor performance much more similar to early APDS rounds than what “WW2 Ballistics: Armor and Gunnery” states. This might be because navy criterion requires set amount of mass to pass through the plate, so penetrations like this weren’t considered? But I’m just throwing ideas out.

[FlipAllTheTables]

@Conraire

90mm T54 Gun

• T44E2 = 3875ft/s or 1181m/s

Wait so the M26E1 should fire APCR at a slightly higher speed?

If so, can a bug report be made on it? Not only the speed but also the name, since in game it’s just called “HVAP shot”. I feel like the ammunition names of the M26E1 could do with some love.

(Edit: I also am interested in the source since I always like expanding my library of knowledge)

[Peasant_wb]

@Conraire Take a look at this: https://apps.dtic.mil/sti/pdfs/ADA953510.pdf

[MiseryIndex556]

If Conraire is using the document I believe he’s using, it lists the T15 and T54 firing the T44 at 3875 fps and the T50 at 3300 fps.

[Conraire]

T44 would still be 3750ft/s, it appears T44E2 uses a different propellant, as it seems to reflect that in the form factors document as well.

[MiseryIndex556]

Interesting. That tan document shows T44 and T44E2 at 3875 fps.